8.4 Manipulate[]

(工事中)

熱方程式の基本解

$$G(x,t)=\frac{1}{\sqrt{4\pi t}}\exp\left(-\frac{x^2}{4t}\right)$$

について、$t$ を固定するごとに $x\mapsto G(x,t)$ のグラフを描いてみる。

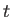
G[x_, t_] := Exp[-x^2/(4 t)]/(2*Sqrt[Pi*t])
g=Plot[Table[G[x, t], {t, 0.1, 1.0, 0.1}], {x, -5, 5}, PlotRange -> All]
Manipulate[Plot[G[x, t], {x, -5, 5}, PlotRange->{0, 3}], {t, 0.01, 2}]

これで $t=0,0.01,0.02,\cdots,2$ の場合の $x\mapsto G(x,t)$ のグラフが描けるが、 再生ボタンを押すことでアニメーションとして見ることができる。

Animate[Plot[G[x, t], {x, -5, 5}, PlotRange->{0, 3}], {t, 0.01, 2}]

としても良い。また

anim=AnimationVideo[Plot[G[x, t], {x, -5, 5}, PlotRange->{0, 3}], {t, 0.01, 2}]
Export["heat.mp4", anim]

として、動画ファイルを作ることもできる。

図 27: 一度に全部描いてしまうと分かりにくい…

離心率が $e$ の円錐曲線は適当な座標系で

$$r=\frac{1}{1+e\cos\theta}$$

と表すことが出来る。

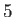
Manipulate[g=PolarPlot[1/(1 + eps Cos[t]), {t, 0, 2 Pi}], {eps, 0, 2, 0.01}]

図 28: $e=0.84$ は楕円