Next: C..4 2次元熱方程式 陰解法 Up: C. 応用: 熱方程式の初期値境界値問題 Previous: C..2 1次元熱方程式 陰解法
2次元の正方形領域
![]() における熱方程式の
初期値境界値問題
における熱方程式の
初期値境界値問題
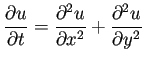 ( (
| ||
アルゴリズムは標準的なもので、どこでも見られるが、例えば 「熱方程式に対する差分法 I」 に説明がある。
まず可視化を gnuplot で行うバージョンを示す。
# heat2d_e_gnuplot.jl --- 空間2次元熱方程式の初期値境界値問題 (Dirichlet,Euler)
#=
using Printf # @printf
using LinearAlgebra # norm()
include("heat2d_e_gnuplot.jl")
heat2d_e_gnuplot(0.05,0.0005,100,50,0.5)
80.647537 seconds (18.70 M allocations: 442.070 MiB, 0.12% gc time)
=#
# 初期値
function u0(x,y)
sin(5 * pi * x) * sin(3 * pi * y)
end
# gnuplot で u を描画
function drawgraph(t, x, y, u, gp)
m,n=size(u)
@printf(gp, "splot '-' with lines title \"t=%.3f\"\n", t)
for i=1:m
for j=1:n
@printf(gp, "%f %f %f\n", x[i], y[j], u[i,j])
end
@printf(gp, "\n")
end
@printf(gp, "e\n")
flush(gp)
end
function heat2d_e_gnuplot(tMax=0.05, dt=0.0005, Nx=100, Ny=50, lambda=0.5)
a = 0.0; b = 2.0; c = 0.0; d = 1.0
hx = (b - a) / Nx; hy = (d - c) / Ny; hx2 = hx * hx; hy2 = hy * hy
tau = lambda * (hx2 * hy2) / (hx2 + hy2)
lambdax = tau / hx2; lambday = tau / hy2
nMax = Int(ceil(tMax / tau))
nskip = max(Int(round(dt / tau)),1)
#
println("tMax=$tMax, Δt=$dt, Nx=$Nx, Ny=$Ny, λ=$lambda")
println("hx=$hx, hy=$hy, tau=$tau")
println("減衰率=e^{-2π^2 τ}=$(exp(-2*pi*pi*tau))")
println("lambda=$lambda, lambdax+lambday=$(lambdax+lambday)")
println("nskip=$nskip")
# メモリー確保
x = range(a, b, length = Nx+1)
y = range(c, d, length = Ny+1)
u = u0.(x*ones(Ny+1)', ones(Nx+1)*y')
newu=zeros(size(u))
# gnuplot の起動
println("gnuplot")
p=open(pipeline(`gnuplot --persist`; stderr=Pipe()), "r+")
println(p.in, "set xlabel \"x\"")
println(p.in, "set ylabel \"y\"")
println(p.in, "set zlabel \"u\"")
println(p.in, "set title \"2D heat equation u_t=u_{xx}+u_{yy}\" at screen 0.4,0.95");
println(p.in, "set xrange [a:b]")
println(p.in, "set yrange [c:d]")
println(p.in, "set zrange [-1.0:1.0]")
println(p.in, "set hidden3d")
println(p.in, "set contour")
# 初期値
t=0.0
drawgraph(t,x,y,u,p.in)
@printf("||u(・,%g)||=%g\n", t, norm(u))
sleep(3)
# 時間発展
for n=1:nMax
t=n*tau
newu[2:end-1,2:end-1]=((1.0-2.0*lambda)*u[2:end-1,2:end-1]
+ lambdax*(u[1:end-2,2:end-1]+u[3:end,2:end-1])
+ lambday*(u[2:end-1,1:end-2]+u[2:end-1,3:end]))
u=copy(newu)
@printf("||u(・,%g)||=%g\n", t, norm(u))
if n % nskip == 0
drawgraph(t,x,y,u,p.in)
end
end
close(p)
end
| ターミナルで |
$ curl -O http://nalab.mind.meiji.ac.jp/~mk/misc/20191229/heat2d_e_gnuplot.jl
$ julia
julia> using Printf
julia> using LinearAlgebra
julia> include("heat2d_e_gnuplot.jl")
julia> heat2d_e_gnuplot()
|
差分方程式を
newu[2:end-1,2:end-1]=((1.0-2.0*lambda)*u[2:end-1,2:end-1]
+ lambdax*(u[1:end-2,2:end-1]+u[3:end,2:end-1])
+ lambday*(u[2:end-1,1:end-2]+u[2:end-1,3:end]))
u=copy(newu)
|
for i=2:Nx
for j=2:Ny
newu[i,j] = ((1.0-2.0*lambda) * u[i,j]
+ lambdax * (u[i+1,j]+u[i-1,j]) + lambday * (u[i,j+1]+u[i,j-1]))
end
end
for i=1:Nx+1
for j=1:Ny+1
u[i,j]=newu[i,j]
end
end
|
次に Plots を利用したバージョン。
| ターミナルで |
$ curl -O http://nalab.mind.meiji.ac.jp/~mk/misc/20200101/heat2d_e_plots.jl
$ julia
julia> using Printf
julia> using Plots
julia> gr()
julia> using LinearAlgebra
julia> include("heat2d_e_plots.jl")
julia> heat2d_e_plots()
|
plot() に渡すとき、 u を転置した u' を渡していることに注意 (' は Hermite 共役を意味するので、 transpose(u) とするのが本当かもしれない)。 転置するのが嫌ならば、初期値の設定を
u=u0.(ones(Ny+1)*x',y*ones(Nx+1)') |
newu[2:end-1,2:end-1]=((1.0-2.0*lambda)*ut[2:end-1,2:end-1]
+ lambdax*(ut[2:end-1,1:end-2]+ut[2:end-1,3:end])
+ lambday*(ut[1:end-2,2:end-1]+ut[3:end,2:end-1]))
u=copy(newu)
|