


Next: 4 Mathematica で音を扱う
Up: Mathematica で Fourier 解析
Previous: 2 Mathematica で Fourier
Mathematica の関数 Fourier[] は、
c=Fourier[f] とすると、
数値のリスト f
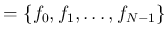 に対して
に対して
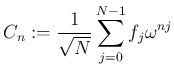
(
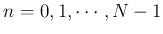
)
を並べたリスト c
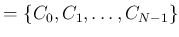 を返す
(これが Mathematica の離散 Fourier 変換の定義)。
逆変換は InverseFourier[c] で行なう。
を返す
(これが Mathematica の離散 Fourier 変換の定義)。
逆変換は InverseFourier[c] で行なう。
可能な場合は高速 Fourier 変換を使って計算されるので、
効率が良くなる (効率をあげたいときは 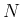 の値に注意すべきである)。
の値に注意すべきである)。
この講義の離散 Fourier 変換の定義に一致する結果を得るには、
次のようなオプションを指定する。
|
Fourier[f,FourierParameters->{-1,-1}]
|



Next: 4 Mathematica で音を扱う
Up: Mathematica で Fourier 解析
Previous: 2 Mathematica で Fourier
桂田 祐史
2016-01-14
![]() に対して
に対して
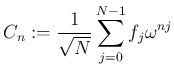 (
(
![]() の値に注意すべきである)。
の値に注意すべきである)。